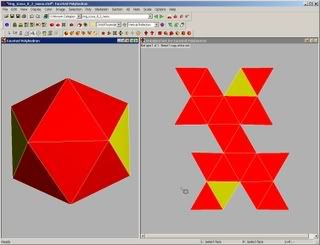
My model, if you were to try it yourself with eight icosahedra, is connected by the faces.
In fact, in my Bryce 5, I was trying to do the math to rotate and position them correctly, and have only gotten a little ways along. I was hoping that once I oriented the first one properly, I could use the "multi-replicate" feature to generate the other seven automagically, rotating them as it is generating. There are 30-degree angles, 45-degree angles, and who knows what else?
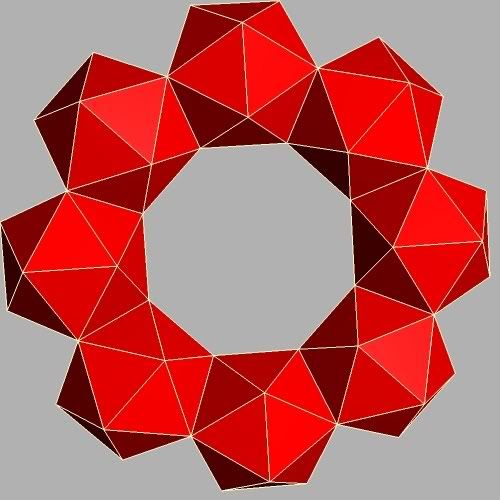
In attempting to cut the preset Stewart toroid, I was aiming for the edges next to the two icosahedra on the right and left; the ones that don't conjoin to the like face that the other ones do. That's funny that they wouldn't have continued the pattern.
I don't know enough about geometry to call all these edges and faces by their values, but don't you see how the face that you must choose to join one to the next is the same set of faces for each one? That the symmetry is obvious, and the asymmetry of their choice in the preset is also obvious?
I'm very curious how you got to the point that you did, and why it wouldn't come around full circle. When I confronted George W. Hart with this problem, he cryptically warned me that this was not an "exact" arrangement; and I don't know how to ponder that.
It would seem to me, since the structure is so beautifully whole, that there must be exact math to its make-up. I suspect somewhere in the formula, there is a margin of error - "flex" - that makes it happen.
It is magical though, don't you think?
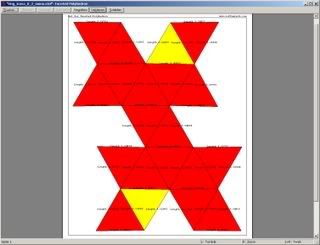
Now, the really cool thing is that I've discovered how to dissect the thing so that it can be made from one piece. That's all I'm going to say about that for now, as I have huge plans. If anyone is interested, I'd entertain business partners.

Rex Everyhting